Trey Turner and Mark Damery, !%REO%!
Advances in production make aspheres more readily available and usable at shorter wavelengths, but the methodology is distinctly different from that of spherical optics. It is vital to understand the cost impact of various specifications — and to communicate top priorities. An experienced asphere manufacturer can propose minor changes to reduce cost significantly while still delivering target performance or better.
Aspheres give the designer a powerful tool that can help reduce the total number of components in a system by decreasing the optical aberrations contributed by each lens surface. For this reason, they are often found in applications that demand that the physical weight and size of the system be minimized, but that still require a high level of optical performance.
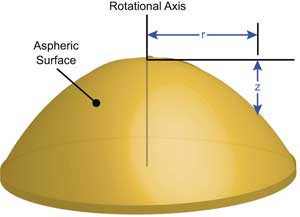
Definition of the aspheric surface variables: r = radial distance from the vertex; z = sag height. Courtesy of REO Inc.
However, although aspheres can make the designer's task easier, they are generally more difficult to fabricate than spherical surfaces and, hence, more costly. Understanding how single-point diamond-turned aspheric lenses for infrared and visible uses are fabricated and tested can help the purchaser properly specify aspheres and avoid creating requirements that drive up cost unnecessarily.
Specifying, fabricating aspheres
The shape of a spherical surface can be specified with a single number: the radius of curvature. In contrast, rotationally symmetric aspheric surfaces are most typically specified using a limited form of the general aspheric equation (Equation 1).
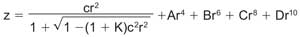
Equation 1. z = sag height; r = radial distance from the vertex; c = base curvature (1/base radius); K = conic constant (0 = sphere, —1 = parabola, ≤1 = hyperbola); A, B, C & D = aspheric coefficients.
This equation may appear in slightly different forms, depending upon the exact sign convention used, so the designer should perform a reality check with the lens manufacturer to ensure that there are no misunderstandings. It is better to have the customer specifically state whether the curve is convex or concave, rather than simply rely on the sign of the curvature term in the equation to indicate shape. The designer should also provide a table of sag heights as a function of radial distance for the curve. This is useful because sag height is the quantity that is most easily measured directly during component fabrication.
Manufacturing tolerances for spherical surfaces are usually stated as power and irregularity values. Power is the amount that the average base radius of curvature deviates from the desired value, and irregularity is how much the part departs from its ideal shape (for example, a perfect sphere). Similarly, aspheres are specified with a tolerance on the base radius (analogous to power), plus a tolerance for sag deviation (equivalent to irregularity). The radius tolerance usually is given as a percentage, with ±0.1 percent being a typical value. A given percentage tolerance on radius becomes progressively more difficult to achieve as the absolute value of the radius gets smaller. As with irregularity, sag deviation is usually stated in (peak to valley) fringes, with a value of 0.5 fringes being typical.
High-precision aspheric lenses for infrared and, in some cases, even visible applications are often fabricated using single-point diamond-turning technology. However, to minimize time, the process often starts with production of a spherical surface that most closely matches the desired asphere. This is accomplished using the same type of generating equipment used for traditional spherical optics fabrication.

Single-point diamond turning of aspheric components is performed one part at a time on a lathe.
To produce the aspheric profile, single-point diamond turning is performed on a lathe configured very much like that used for machining metal parts, albeit built to achieve much higher precision. The cutting tools themselves are gem-quality diamonds. The addition of multiaxis tool motion synchronized with spindle rotation also enables the production of nonrotationally symmetric surfaces such as toroids and off-axis parabolas.
The initial setup for diamond turning involves centering the part on the spindle and setting several process variables, such as tool angle, tool location, depth of cut, feed rate, position of the coolant, and even the ambient temperature and humidity in the room. This process is time-consuming and thus costly. However, once the equipment is properly configured, it is possible to produce many parts without having to make major adjustments. As a result, diamond turning is most cost-effective when used with medium- to high-production volumes.
The use of diamond turning also opens up the possibility of directly producing a diffraction grating profile on the surface of an optic. This can be a particularly powerful tool for the designer, enabling the correction of various wavefront errors as well as making it possible to achromatize an element using only a single material.
However, just as in the case of aspheric surfaces themselves, there is no universal standard on how diffractive surfaces are mathematically described. Thus, it is important to communicate all the relevant parameters of the grating to the manufacturer. These include the diffractive coefficients, the refractive index of the materials preceding and following the grating surface, the diffractive order, the profile direction and the number of zones required.
Fabrication cost factors
The single-point diamond-turning fabrication technology used to produce aspheres has certain distinct characteristics, advantages and limitations. Understanding these can help the buyer to obtain components that perform well, yet still minimize costs.

Single-point diamond turning can be used on a variety of materials and can produce features such as through holes, mounting flanges and even grating profiles.
Of these factors, one of the most important is the different cutting characteristics of various commonly used materials. For example, silicon is difficult to machine and wears cutting tools very rapidly. A tool might cut literally just a few silicon parts before it must be changed out or sharpened, which requires time and therefore incurs cost. CaF2 also wears tools rapidly and typically might yield a dozen or so parts before replacement. In contrast, ZnS and ZnSe are easier to machine, and it's not uncommon to produce two to three times as many parts of the same size with a single tool. Germanium is perhaps the material for the infrared that is softest and easiest to machine; processing hundreds of parts might be possible before tool replacement is required.
Diamond turning generally produces a rougher surface finish than traditional polishing techniques, so it is most commonly employed for infrared optics where a given roughness has less of a performance impact. However, roughness is still an important consideration and is usually specified for diamond-turned parts. But, oddly enough, the tool wear characteristics of materials do not correlate with the level of surface roughness that can be routinely achieved with them. Specifically, for diamond-turned CaF2 parts, a surface roughness in the 50-Å range is typically achieved, while ZnS and ZnSe can be routinely machined to the 40-Å level. Silicon wears tools rapidly but cuts cleanly, and a 15-Å surface roughness value is possible with this material. Germanium is again a standout here, and surface roughnesses as low as 10 Å can be achieved on it. Specifying surface roughness values significantly lower than those enumerated here is likely to drive up production costs substantially.
For all materials, aspect ratio (diameter:thickness ratio) is another important consideration. Components with a high aspect ratio are particularly difficult to produce and tend to have some edge roll-off (an area where the actual shape departs from the desired curve). Typically, the clear aperture (the diameter over which specified tolerances and performance must be maintained) for diamond-turned optics is 90 percent of the part diameter. Specifying a larger clear aperture than this on a component with a relatively high aspect ratio would drive production costs up. In other words, if the shape of the part must be held near to the edge, it's better to make the part thicker.
One advantage of diamond turning is that accommodating features such as through holes or mounting flanges while the part is being turned is fairly easy. However, the production of center holes in particular can drive up fabrication costs because it eliminates the curve vertex that is often used as the reference point for subsequent measurements of surface profile.
Metrology considerations
Because of their shapes, aspheric surfaces typically cannot be measured using exactly the same techniques employed with spherical surfaces. The simplest and least expensive method for gauging aspheric surface shape is with the use of a contact profilometer. These instruments operate by drawing a small stylus over the surface and measuring its up-and-down movement. Each individual measurement is performed along only a single line, so measurements at multiple different azimuthal angles may sometimes be required to build up a three-dimensional picture of part shape.
Laser interferometers, which are used almost universally for testing spherical optics to very high degrees of precision, also can be adapted for measuring aspheres, usually with the addition of correction optics (called null optics) that suitably modify the wavefront. This is typically accomplished with a computer-generated hologram (CGH) specifically designed to make the necessary wavefront corrections for the particular asphere under test.
The drawback of this approach is cost: A CGH might cost $10,000 or more and is useful for testing only one specific aspheric profile. However, interferometric measurements with a CGH provide a map of the entire surface, not just along a single line or lines, and can typically be performed more rapidly than surface profilometry (once initial interferometer setup is complete). Thus, interferometric testing may be a more economical alternative for high-volume production.
Interferometric testing is sometimes virtually a necessity with certain types of parts. For example, high-aspect-ratio parts will often exhibit some astigmatism after they are removed from the chuck. While this can be identified by using a sufficient number of line measurements with surface profilometry, it is far more readily characterized by interferometric testing with a CGH. Similarly, aspheric profiles that depart substantially from a sphere, or those that do not have rotational symmetry, are more easily measured with interferometry.

Optical profilometers enable accurate characterization of surface roughness but operate over only a small field of view.
As mentioned previously, surface roughness tends to be a more significant issue with aspheric surfaces and must be measured in any quality control/quality assurance protocol for aspheres. Larger values for surface roughness (100 Å and above) can be measured with a stylus profilometer, but accurately characterizing surfaces below this level is generally accomplished using an optical profilometer. These are microscope-based instruments that deliver extremely accurate measurements. However, they capture only a small field of view at one time, so it's generally not possible to measure the entire surface of an optic. Therefore, the purchaser should designate the microscope magnification at which measurements are to be performed as well as the number and location of the sampling points. For example, "measure at 10x at three points (center, middle and edge) on each part."
Optical profilometers have a limited depth of focus, which can make it difficult to properly visualize the entire field of view when looking at particularly steep parts, such as off-axis parabolas. In this case, special fixturing might be required to precision-tilt the part so that proper focus can be obtained across the entire field of view, and this incurs additional cost.
Meet the authors
Trey Turner is chief technology officer at REO; e-mail: treyt@reoinc. com. Mark Damery is REO's vice president and general manager of worldwide sales; e-mail: [email protected].