Dr. Ulrike Fuchs and Sven R. Kiontke, Asphericon GmbH
Afocal beam expansion systems based on the basic principles of Kepler and Galileo telescopes can be improved in terms of wavefront quality by using additional optical surfaces or aspherizing them using monolithic beam expansion elements.
Everyday work in an optics laboratory would be unthinkable without beam expansion systems, which optimally adjust beam cross sections between the light sources (e.g., lasers) and the subsequent optical elements. Precise illumination of the optically effective surfaces is essential, especially for beam shaping and focusing with high numerical apertures. The most widespread basic principles of afocal beam expansion systems are based on the telescopes of Kepler and Galileo. This article will reveal various modes of action in more detail, and discuss new approaches based on the use of aspherical surfaces.
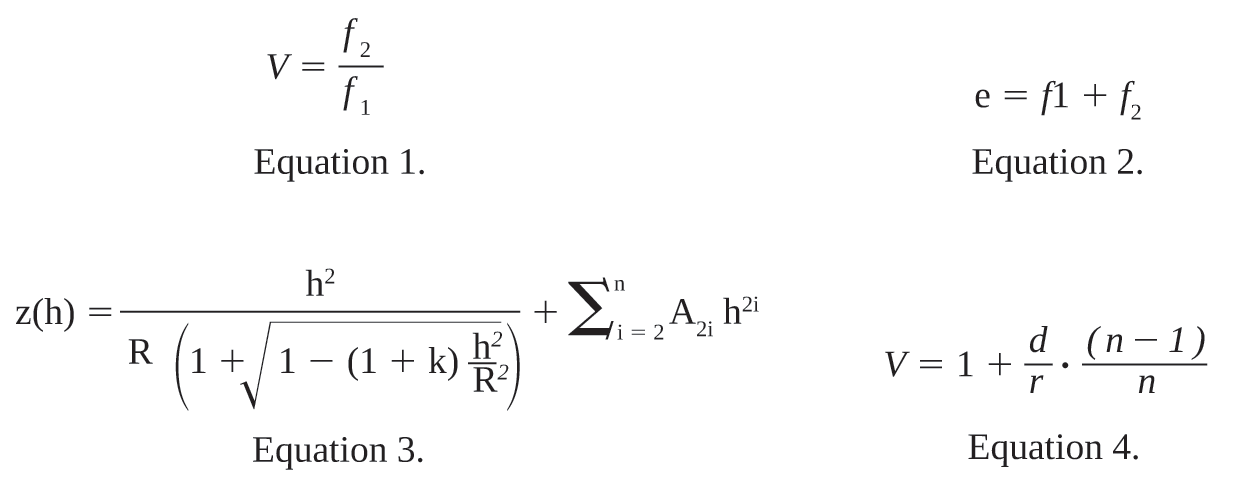
The simplest principle of a beam expansion system is the combination of two convergent lenses with different focal lengths, corresponding to the configuration of a Kepler telescope. The enlargement or reduction of the beam cross section results from the relationship between the focal lengths as per Equation 1. The total length of this optical system is primarily determined by the distance between the lenses, which can be estimated using the sum of the focal lengths (Equation 2).
If the construction length needs to be shortened while retaining the enlargement, the first convergent lens (Figure 1, left) can be replaced with a divergent lens, resulting in a construction similar to that of a Galileo telescope.
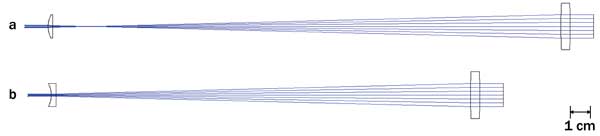
Figure 1. Illustration of the course of a beam for a Kepler (a) and a Galileo (b) telescope for 10× enlargement, realized with a convergent lens with a long focal length (f2 = 200 mm) and a convergent (f1 = 20 mm) (a) or divergent lens (f1 = –20 mm) (b) with a short focal length.
In addition, the sign of the enlargement changes, whereby the beam profile is no longer subject to point reflection during enlargement. Figure 1 shows both a Kepler (a) and a Galileo (b) telescope for 10× enlargement, realized with a convergent lens with a long focal length (f2 = 200 mm), and a convergent (f1 = 20 mm) or divergent lens (f1 = –20 mm) with a short focal length. To further shorten the construction length while retaining the enlargement, according to Equations 1 and 2, focal lengths f1 and f2 should be scaled correspondingly with a factor. The same enlargement of M = 10, for instance, also can be achieved with the focal lengths f1 = 150 mm and f2 = 15 mm. If only spherical lenses are used in this kind of configuration, spherical aberrations soon become noticeable, reducing the quality of the wavefront for the expanded beam. Figure 2a shows the resultant wavefront as a cross section.
This comparison clearly shows the compromise between the achievable wavefront quality and minimal achievable construction length when using spherical lenses – with a certain minimal requirement on the quality of the wavefront, it is not possible to go below a certain construction length using this mode of action.
The use of achromatic groups counteracts this effect (Figure 2b). This shows a Galileo telescope for the same enlargement M = 10, in which a doublet with f2 = 150 mm has replaced the convergent lens. The spherical aberrations can thus be reduced significantly while retaining the construction length. This effect can be increased further by adding additional optical surfaces.
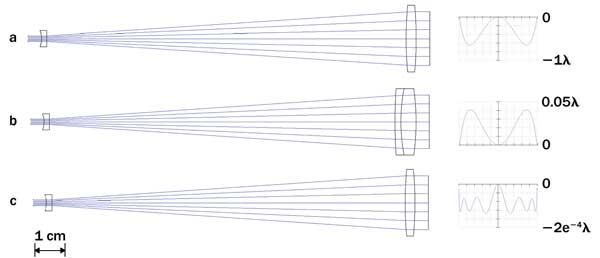
Figure 2. Illustration of three implementations of a Galileo telescope for the enlargement M = 10, realized using the focal lengths f1 = 150 mm and f2 = 15 mm, with (a) a spherical convergent lens, (b) an achromatic doublet and (c) an aspherical convergent lens. The gradual reduction of the wavefront aberrations by several orders of magnitude is very easy to understand using the cross-section images on the right.
An alternative approach is to aspherize one of the lens surfaces, whereby the spherical aberrations can be reduced to a minimum in line with the working principle. The shape of the lens surface differs from that of a sphere and can be described by Equation 3.
In Figure 2c, such a beam expansion system for M = 10 and f2 = 150 mm is shown in comparison to the approach using an achromatic lens. In the selected example, the last optical surface (on the right) has an aspherical design.
If this kind of system is used for beam expansion, the shape of the optical surfaces and the lens spacing are usually optimized for one wavelength (or, if using a doublet, two). Because of this, using the system for another wavelength inevitably results in greater wavefront error and additional divergence. By slightly altering the spacing of the lenses, the afocal system can be reproduced, minimizing the residual divergence and the spherical aberrations. Occasionally, a targeted misalignment of the formerly afocal system is also desirable to produce or compensate for a defined residual divergence.
Monolithical beam expansion systems take a slightly different approach. In terms of the mode of action, they correspond to the Galileo telescope, although they consist of only one optical element – a meniscus lens, which means both of the optically effective surfaces possess a common center of curvature. The principle behind this has already been known for some time, although they produce severe spherical aberrations in their original design with two spherical surfaces and thus can be used for only very small incoming beam diameters and very small enlargements. Figure 3 shows an example of such a lens.
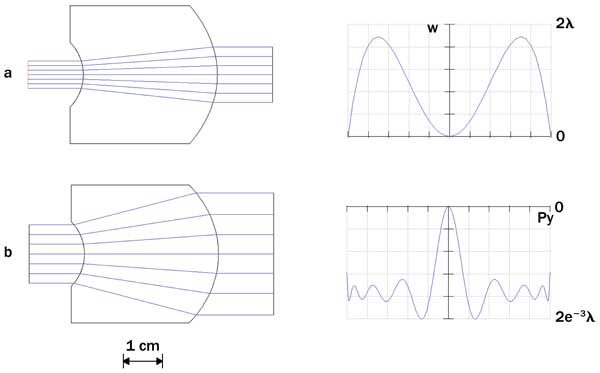
Figure 3. Two monolithic beam expansion systems are shown, (a) with spherical surfaces and (b) with a (convex) aspherical surface, for an enlargement of M = 2. The incoming beam diameter is 5 mm in (a) and 10 mm in (b). Nevertheless, the resulting wavefront errors for the aspherical solution are three times smaller.
These optical elements become very interesting when one of the two surfaces is aspherized. In line with the mode of action, this enables the spherical aberrations to be corrected and an afocal system to be realized, even for large incoming beam diameters. The improvement in the optical properties is clearly visible in the comparison in Figure 3. The enlargement corresponds in both cases to M = 2, whereby the incoming beam diameter is decreased by a factor of two.
One of the most exciting questions in this context is, of course: How large is the maximum enlargement that can be achieved with such an individual element? This is estimated using the paraxial enlargement (Equation 4), whereby n is the refractive index of the glass, r the radius of the concave side and d the center thickness. If glass is chosen as the material, the refractive index in VIS is 1.4 <n <2.1 and, therefore, the corresponding factor is approximately between 0.3 and 0.5. Accordingly, the contribution of the two summands is significantly determined by the relationship between the center thickness d and radius of curvature r of the concave surface.
Of course, in principle, a very large center thickness could be chosen, but this does not make practical sense. As a result, a limit is set on an aspect ratio of center thickness to diameter of 1 (e.g., center thickness = diameter = 25 mm) for this estimation. The concave radius also has a lower limit of approximately 8 mm; a smaller radius would place significant unnecessary limitations on the free aperture of the lens. This produces an optimum in the maximum individual element enlargement of M = 2. If these considerations are extended to semiconducting materials for use in IR, enlargements of up to M = 3.5 are possible.
As shown, the individual element enlargements of monolithic Galileo telescopes are relatively small as a result of the limitation in center thickness. However, as these are afocal beam expansion systems, they can be connected in series to successively enlarge the incoming beam one after the other in the beam course (Figure 4).
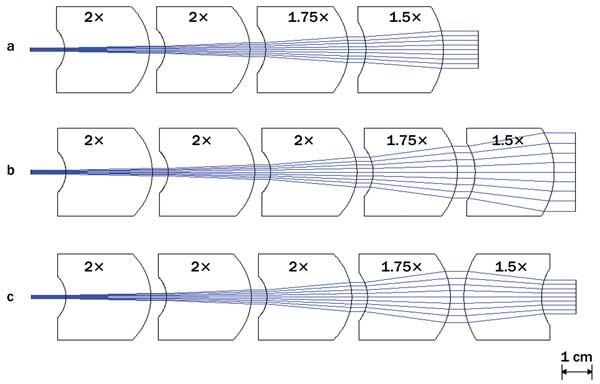
Figure 4. Illustration shows cascade systems for beam expansion based on monolithic individual systems: (a) 10.5× enlargement, (b) 21× enlargement and (c) 9.3× enlargement. The systems (a) and (b) differ by the additional element with M = 2. When transferring from (b) to (c), the orientation of the last element with M = 1.5 has been inverted.
This opens up new opportunities. Just three of these elements can enlarge the beam by eight times; five elements, 32 times. If only individual elements with M = 2 are used, the increments of the possible enlargements are very approximate at M = 2, 4, 8, 16, 32 ... . This is an optimal solution if one requires strong enlargement with minimal space used and a high wavefront quality.
If, however, finer increments between the enlargement levels are desired, it is necessary to introduce other individual element enlargements, which also lie very close together. Two lower levels are offered here at M = 1.5 and M = 1.75, especially for the version in glass. Because of the afocal dimensioning of the individual elements, the meniscus lenses can be oriented in the course of the beam however one likes, as shown in Figure 4c. This means, when combined, there are not only three but actually six individual element enlargements available, which significantly increases the combinatorics level. If, for instance, one element is available for each basic enlargement, this produces 13 possibilities for the overall enlargement with just these three meniscus lenses. If we push these combinatorics further with additional elements, an above-average number of combination options are opened up by certain lens groupings.
What is common to all these groups is the presence of one element each with M = 1.5 and M = 1.75 and an increasing number of elements with M = 2. Figure 4b shows an example of an overall enlargement of M = 21 consisting of five individual elements. Using the specific group shown there (1 × M = 1.5; 1 × M = 1.75; 3 × M = 2), it is possible to realize 62 enlargement levels with the maximum at M = 21.
In practice, to implement such an aspherical cascade system for flexible beam expansion, very high surface qualities for the individual elements are required. To prevent restrictions on combinatorics for later use, each individual element must be significantly better over the whole free aperture than the “diffraction limited” requirement (i.e., wavefront error RMS <λ/14). For a Ti:sapphire laser wavelength at 780 nm, for instance, RMS <55 nm; for λ = 532 nm, it is even just RMS <32 nm. If the center thickness and the decentration of the surfaces are also produced very precisely for these requirements, this system is adjustment-free. This means that the attachment of additional monolithic elements to change the enlargement level also takes place completely adjustment-free and is thus quick and easy.
Meet the authors
Dr. Ulrike Fuchs is head of applications at asphericon GmbH in Jena, Germany; email:[email protected]. Sven R. Kiontke is general manager at asphericon; email: [email protected].